线性表及实现

**在学习浙江大学的数据结构慕课时，发现了一些问题，在本文章中记录并分享给大家。

在多重链表的表示中有这样一个问题：

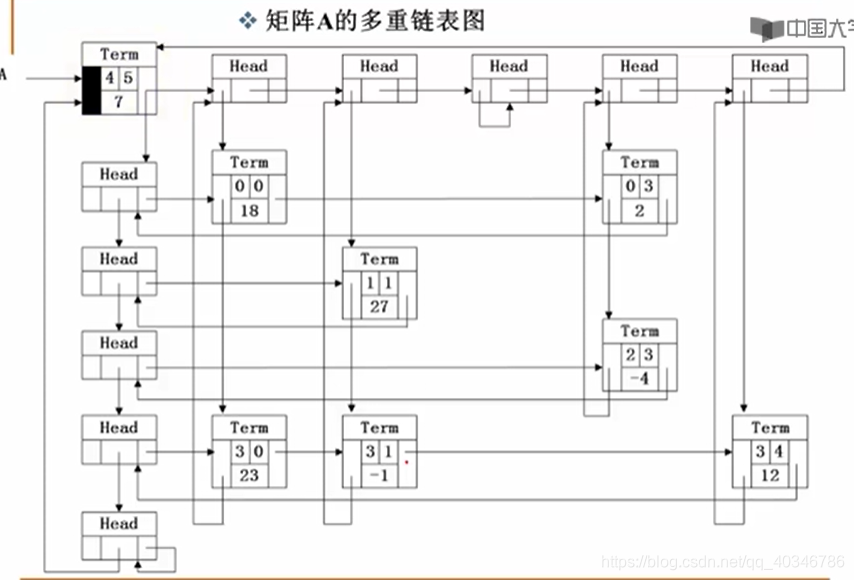
矩阵的多重链表表示

**第i行的head和第i列的head实际上是同一个结点
从图中看见表有一个总的头结点存储矩阵的大小和非零元素的个数，同时指向着每行每列的第一个头结点。但实际上第i行第i列的头结点是同一个头结点。第i行第i列的head存储着下i+1行/列的结点指针，也同时存储着第i行的第一个非零元素和第i列的第一个非零元素。因此上面4X5的矩阵一个有5个头结点。

堆栈

在用单向链表实现堆栈时，必须从头结点指针指向top位置，L->next即top位置，可以自由的增删，而不能从尾部，因为尾部无法进行插入操作。

课后习题中有一个堆栈的问题如下：
原题：
Given a stack which can keep M numbers at most. Push N numbers in the order of 1, 2, 3, …, N and pop randomly. You are supposed to tell if a given sequence of numbers is a possible pop sequence of the stack. For example, if M is 5 and N is 7, we can obtain 1, 2, 3, 4, 5, 6, 7 from the stack, but not 3, 2, 1, 7, 5, 6, 4.

Input Specification:
Each input file contains one test case. For each case, the first line contains 3 numbers (all no more than 1000): M (the maximum capacity of the stack), N (the length of push sequence), and K (the number of pop sequences to be checked). Then K lines follow, each contains a pop sequence of N numbers. All the numbers in a line are separated by a space.

Output Specification:
For each pop sequence, print in one line “YES” if it is indeed a possible pop sequence of the stack, or “NO” if not.

思考了许久没有什么头绪，只想到是否能用数之间的大小关系来判断，如756这样的顺序是肯定不行的，但又无法解决栈空间不够的问题。在网上搜索发现有一位博主的思路很好，链接如下：
原文链接：https://blog.csdn.net/gxtyx/article/details/105327934

手动用数组实现栈来模拟上面的问题，手动出栈入栈，很方便的解决栈空间的问题，对于顺序的判断也很简单快速。
首先需要将1~N的输入存储起来作为栈输入，然后对顺序输出也需要存储来判断出栈的时机，还需要一个数组模拟栈。代码如下：

#include <stdio.h>
/* 02-线性结构4 Pop Sequence (25分) */

#define MAX 1000

int main(void)
{
    int arr[MAX], stack[MAX], input[MAX];
    int m, n, k, ai = 0, si = 0, ii = 0;    /* ai, si, ii分别是数组arr, stack, input的下标 */
    
    scanf("%d %d %d", &m, &n, &k);
    for(int i = 1; i <= n; i++) /* 按题目要求生成一个入栈顺序数组1到N */
        arr[i-1] = i;
    for(int j = 0; j < k; j++)
    {
        ai = 0;
        si = -1;    /* 这里stack下标初始化为-1是因为在下方进入while循环中会先+1 */
        ii = 0;
        for(int i = 0; i < n; i++)
            scanf("%d", &input[i]);
        while(ai < n)
        {
            si++;
            stack[si] = arr[ai++];
            if (si > m - 1)     /* 判断是否栈满 */
                break;
            while(stack[si] == input[ii] && si >= 0)    /* 出栈 */
            {
                si--;
                ii++;
            }
        }
        if (si > m - 1 || ii != n)
            printf("NO\n");
        else
            printf("YES\n");
    }
    
    return 0;
}