[数据结构与算法]leetcode2017网格游戏（中等，周赛）

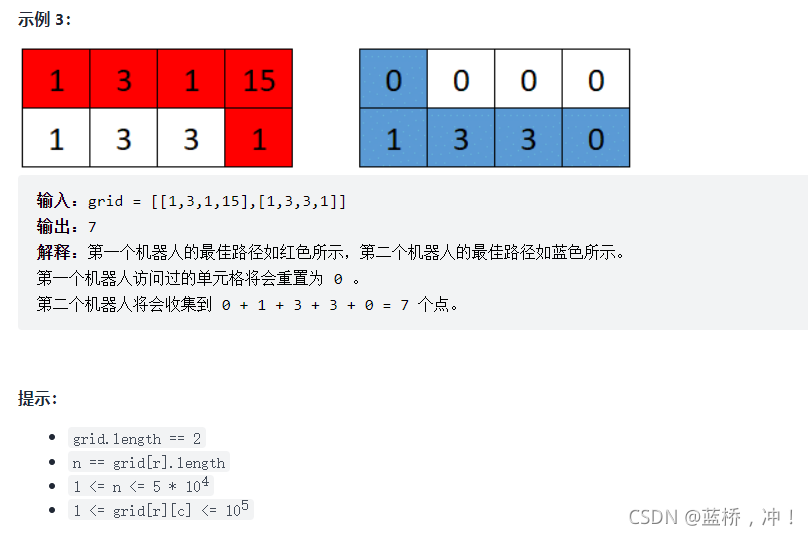
思路：
第一个机器人移动的路径是一个 一丨一（像Z），所以第二个机器人想要收集的点数最多，也就是取右上方和坐下方的较大值，即：第一个机器人找出一个下标index，使得max(grid[1][index]左边的和，grid[0][index]右边的和)最小。

class Solution {
public:
    long long gridGame(vector<vector<int>>& grid) {

        int n = grid[0].size();
        //先得到grid 从0到grid[0].size()-1的前缀和pre[]
        //先得到grid 从grid[1].size()-1到0的后缀和post[]
        vector<long long> pre(n);
        vector<long long> post(n);
        post[n - 1] = 0;
        pre[0] = 0;
        for (int i = n - 2; i >= 0; --i) {
            post[i] = post[i + 1] + grid[0][i + 1];
        }
        for (int i = 1; i < n; ++i) {
            pre[i] = pre[i - 1] + grid[1][i - 1];
        }
        
        long long m = ~(1LL << 63);
        for (int i = 0; i < n; ++i) {
            if (max(pre[i], post[i]) < m) {
                m = max(pre[i], post[i]);
            }
        }
        return m;  
    }
};