开发: C++知识库 Java知识库 JavaScript Python PHP知识库人工智能区块链大数据移动开发嵌入式开发工具数据结构与算法开发测试游戏开发网络协议系统运维
教程: HTML教程 CSS教程 JavaScript教程 Go语言教程 JQuery教程 VUE教程 VUE3教程 Bootstrap教程 SQL数据库教程 C语言教程 C++教程 Java教程 Python教程 Python3教程 C#教程
数码: 电脑笔记本显卡显示器固态硬盘硬盘耳机手机 iphone vivo oppo 小米华为单反装机图拉丁

-> 数据结构与算法 -> 详解二叉树的三种遍历方式（递归、迭代、Morris算法） -> 正文阅读

[数据结构与算法]详解二叉树的三种遍历方式（递归、迭代、Morris算法）

详解二叉树的三种遍历方式（递归、迭代、Morris算法）

最重要的事情写在前面：遍历顺序不一定就是操作顺序！！！

递归解法

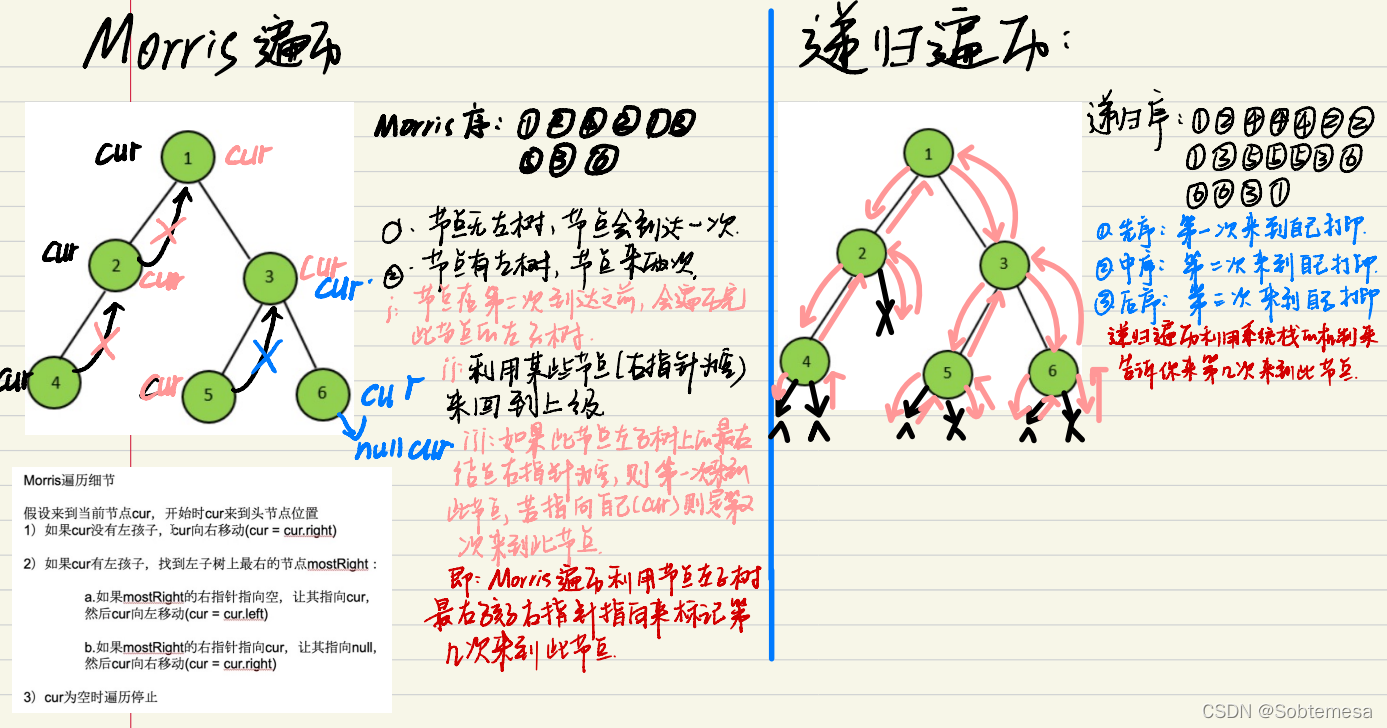
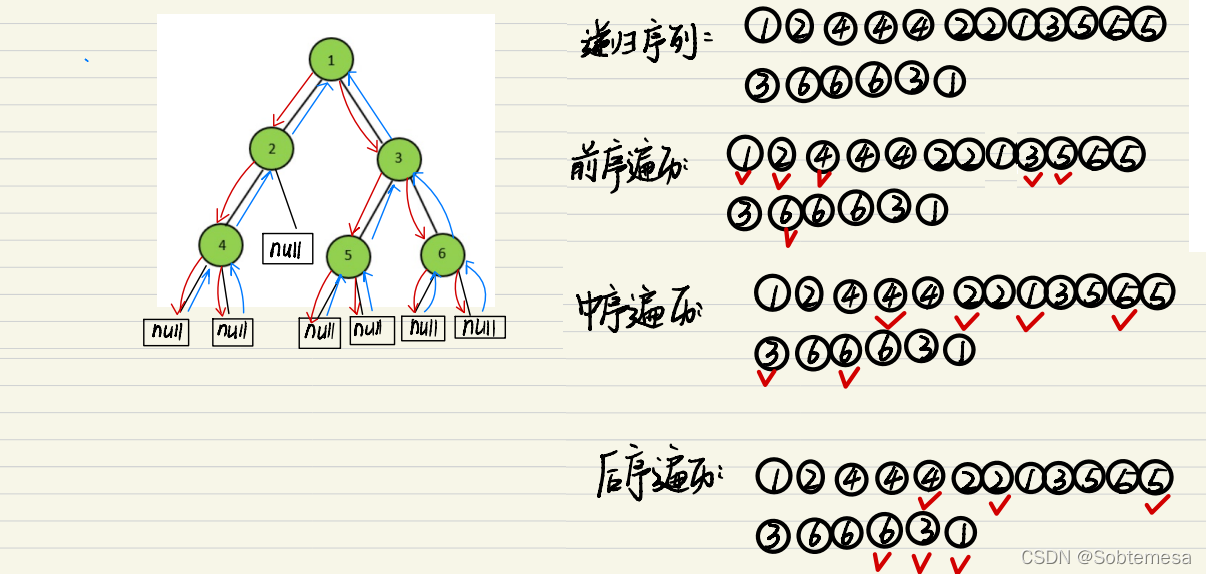
首先，一颗二叉树它的递归序列是一定的，导致其前中后序不同的原因只不过是访问节点的时机不同。

递归序列：1 2 4 4 4 2 2 1 3 5 5 5 3 6 6 6 3 1

a:先序：第一次来到自己打印。1 2 4 3 5 6

b:中序：第二次来到自己打印。4 2 1 5 3 6

c:后序：第三次来到自己打印。4 2 5 3 6 1

在这里插入图片描述

代码：（前序–中序–后序）

/**
 * 前序遍历递归
 * @param root
 */
   public void preOrder(BTNode root){
   if(root==null){
      System.out.print("NULL->");
      return;
   }
   //第一次来到此节点
   System.out.print(root.val+"->");
   preOrder(root.left);
   preOrder(root.right);
   }

/**
 * 中序遍历递归
 * @param root
 */
public void InOrder(BTNode root){
   if(root==null){
      System.out.print("NULL->");
      return;
   }

   InOrder(root.left);
   //第二次来到此节点
   System.out.print(root.val+"->");
   InOrder(root.right);
}

/**
 * 后序遍历递归
 * @param root
 */
public void PostOrder(BTNode root){
   if(root==null){
      System.out.print("NULL->");
      return;
   }

   PostOrder(root.left);
   PostOrder(root.right);
     //第三次来到此节点
   System.out.print(root.val+"->");
}

迭代解法

递归的实现就是：每一次递归调用都会把函数的局部变量、参数值和返回地址等压入调用栈中，然后递归返回的时候，从栈顶弹出上一次递归的各项参数，所以这就是递归为什么可以返回上一层位置的原因。
此时大家应该知道我们用栈也可以是实现二叉树的前后中序遍历了。
递归的时候隐式地维护了一个栈，而我们在迭代的时候需要显式地将这个栈模拟出来，其余的实现与细节都相同。

前序遍历：

解法一:

/**
 * 前序遍历迭代
 * @param root
 */
public List<Integer> preOrder3(BTNode root){
   Stack<BTNode> stack=new Stack<>();
   List<Integer> res = new ArrayList<Integer>();
   BTNode cur=root;
   if(root==null){
      return res;
   }
   // cur不为null时入栈，向left方向推进直到叶子节点
   while(cur!=null){
         stack.push(cur);
         res.add(cur.val);
         cur=cur.left;
      }
    while (!stack.empty()){
      // 向left推进到叶子节点后弹出该叶子节点
      BTNode top=stack.pop();
      // 左子树遍历完毕，回到该节点,向right推进.
      cur=top.right;
   }
   return res; 
   }

也可以写成这样：

/**
 * 前序遍历迭代
 * @param root
 * @return
 */
public List<Integer> preOrder3(BTNode root){
   Stack<BTNode> stack=new Stack<>();
   List<Integer> res = new ArrayList<Integer>();
   BTNode cur=root;
   if(root==null){
      return res;
   }
   // 同时满足root为null及stack为空，才将结果返回
   while (!stack.empty()||cur!=null){
      // cur不为null时入栈，向left方向推进直到叶子节点
      while(cur!=null){
         stack.push(cur);
         res.add(cur.val);
         cur=cur.left;
      }
      // 向left推进到叶子节点后弹出该叶子节点
      BTNode top=stack.pop();
      // 左子树遍历完毕，回到该节点,向right推进.
      cur=top.right;
   }
   return res;
}

解法二：

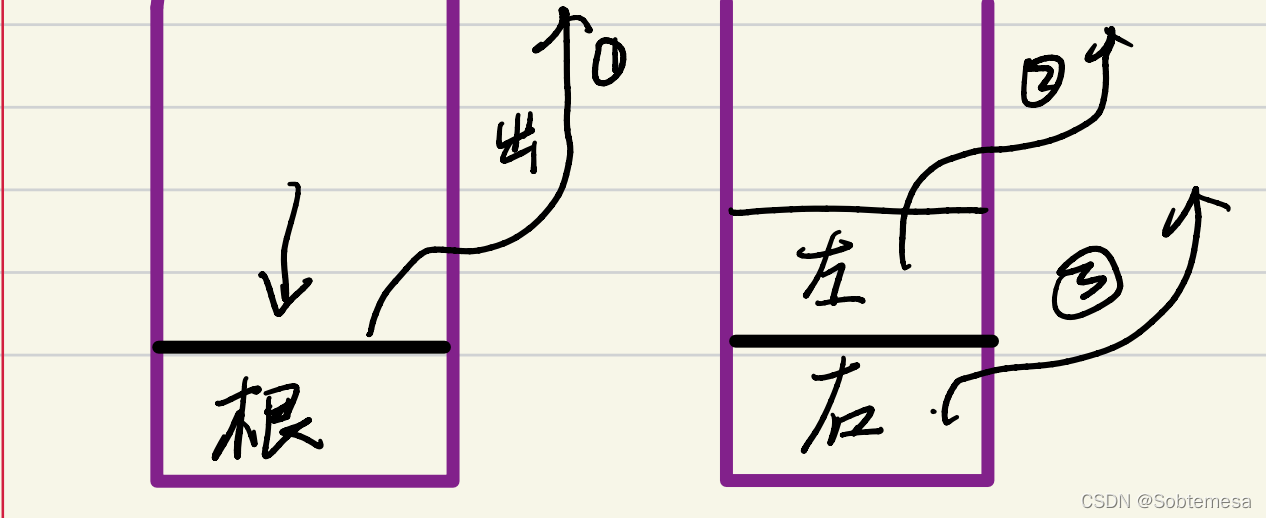
首先我们应该创建一个Stack用来存放节点，首先我们想要打印根节点的数据，此时Stack里面的内容为空，所以我们优先将头结点加入Stack，然后打印。

之后我们应该先打印左子树，然后右子树。所以先加入Stack的就是右子树，然后左子树。
此时你能得到的流程如下:

在这里插入图片描述

/**
 * 前序遍历迭代
 * @param root
 */
public List<Integer> preOrder2(BTNode root){
   Stack<BTNode> stack=new Stack<>();
   List<Integer> res = new ArrayList<Integer>();

   if(root!=null){
      stack.push(root);
   }
   while (!stack.empty()){
      BTNode top=stack.pop();
      res.add(top.val);
      if(top.right!=null)stack.push(top.right);
      if(top.left!=null)stack.push(top.left);
   }
   return res;
}

中序遍历：像递归一样，在前序遍历的基础上稍作修改即可。

/**
 * 前序遍历迭代
 * @param root
 */
public List<Integer> preOrder3(BTNode root){
   Stack<BTNode> stack=new Stack<>();
   List<Integer> res = new ArrayList<Integer>();
   BTNode cur=root;
   if(root==null){
      return res;
   }
   // cur不为null时入栈，向left方向推进直到叶子节点
   while(cur!=null){
         stack.push(cur);        
         cur=cur.left;
      }
    while (!stack.empty()){
      // 向left推进到叶子节点后弹出该叶子节点
      BTNode top=stack.pop();
        res.add(top.val);
      // 左子树遍历完毕，回到该节点,向right推进.
      cur=top.right;
   }
   return res; 
   }

后序遍历：

对于迭代的操作，则后续遍历稍显麻烦。
因为左 -> 右 -> 中的访问过程中当遍历到最左边，如果没有控制好右侧节点的链表指向，可能会造成死循环的问题。
解决问题的关键，当遍历到最左边节点的右子树为空的状态或者右子树已经处理过，需要用pre记录这个节点，表示该节点以及它的子树已经完全处理好了，避免造成栈与链表的循环访问即可。

   /**
    * 后序遍历迭代
    *
    * @param root
    */
   public List<Integer> postOrder2(BTNode root){
      Stack<BTNode> stack=new Stack<>();
      List<Integer> res = new ArrayList<Integer>();
      if(root==null) return res;
      BTNode cur=root;
       //当遍历到右节点为空的状态时，需要记录他的pre节点
      BTNode pre=null;
     
      while (!stack.empty()||cur!=null){
          // cur不为null时入栈，向left方向推进直到粗人为空
         while (cur!=null){
            stack.push(cur);
            cur=cur.left;
         }
          //cur为空，看看该节点有无右子树或者已经处理过右子树
         BTNode top=stack.peek();
         if(top.right==null||top.right==pre){
            //1.top.right==null右树为空，说明为叶子节点，直接添加top.val
             //2.top.right==pre右树已经处理过了，左树为空，右树处理过，直接添加top.val就可以了
            stack.pop();
            res.add(top.val);
             //用pre记录这个节点，表示该节点以及它的子树已经完全处理好了
            pre=top;
         }else {
            cur=cur.right;
         }
      }
      return  res;
   }

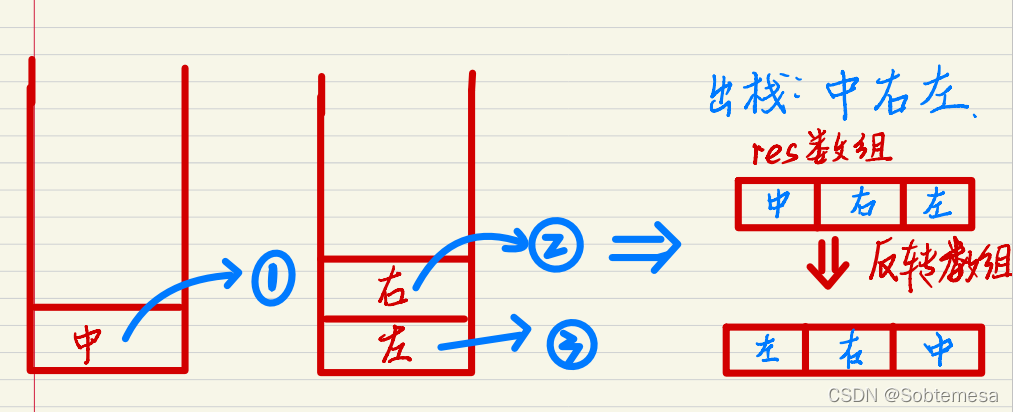
还有一种比较好理解的解法：

先序遍历是中左右，后序遍历是左右中，那么我们只需要调整一下解法二先序遍历的代码顺序，就变成中右左的遍历顺序，然后在反转result数组，输出的结果顺序就是左右中了

在这里插入图片描述

代码：

  public List<Integer> postOrder3(BTNode root){
      Stack<BTNode> stack=new Stack<>();
      List<Integer> res = new ArrayList<Integer>();
      if(root==null) return res;
      BTNode cur=root;
     stack.push(cur);
      while (!stack.empty()){
        BTNode top=stack.pop();
        res.add(top.val);
        if(top.left!=null)stack.push(top.left);
        if(top.right!=null)stack.push(top.right);
      }
      Collections.reverse(res);
      return  res;
   }

Morris算法

有一种巧妙的方法可以在线性时间内，只占用常数空间来实现前、中、后序遍历。这种方法由 J. H. Morris 在 1979
年的论文「Traversing Binary Trees Simply and Cheaply」中首次提出，因此被称为 Morris
遍历。

二叉树的前中后序遍历均涉及到从根出发，向叶子方向推进后再返回的过程。无论是递归方法还是显式地使用栈的迭代方法，能够返回的关键均在于栈。思考如何在不使用栈的情况下实现二叉树遍历时的返回动作。以下图的中序遍历为例，在完成[4, 2, 5]的遍历之后要遍历1(将1加入到结果中)，如果在完成5的遍历后能够通过5的指针信息返回到1，就有可能在不使用栈的情况下完成二叉树的遍历。实际上这就是线索二叉树(Threaded Binary Tree)的思想，而Morris遍历算法正是这种思想的具体应用。

在这里插入图片描述

Morris遍历细节：

假设来到当前节点cur,开始时cur来到头节点的位置

1)如果cur没有左孩子，cur向右移动（cur=cur.right)

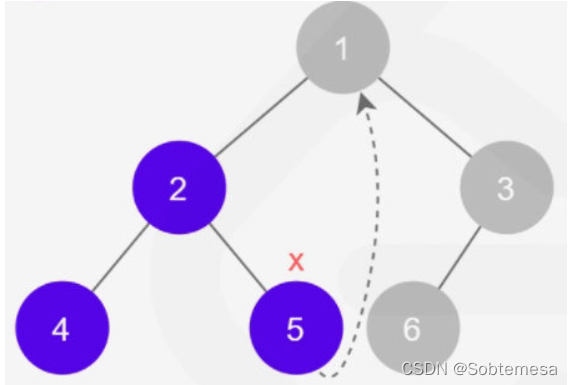
2)如果cur有左孩子，找到左子树上最右的节点pred,即中序遍历下当前节点的前驱节点

? a:如果pred.right==null，置prev=cur,然后cur向左移动（cur=cur.right)

? b:如果pred.right==cur,让其指向null，恢复树形，然后cur向右移动（cur=cur.right)

3）cur==null时遍历结束

图示：

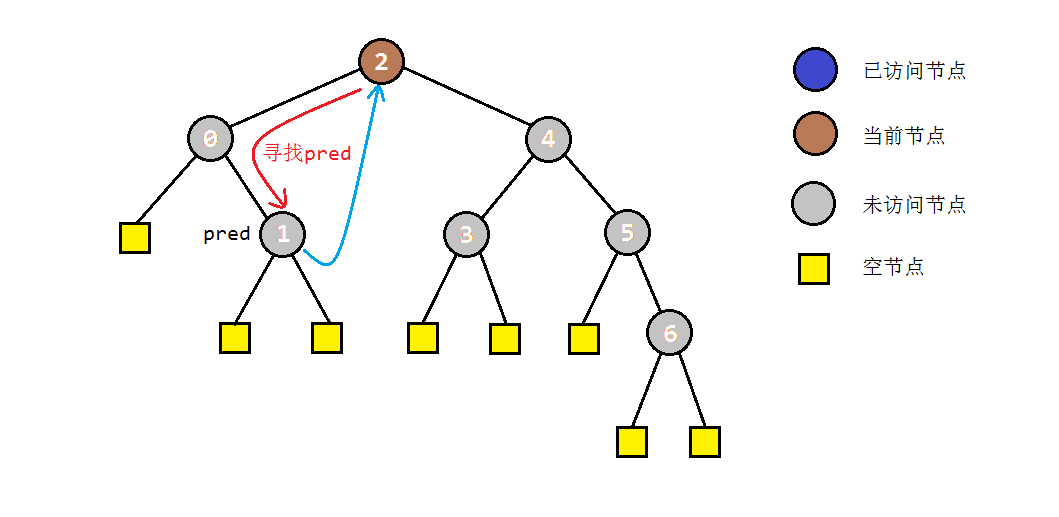
Morris遍历过程1.png

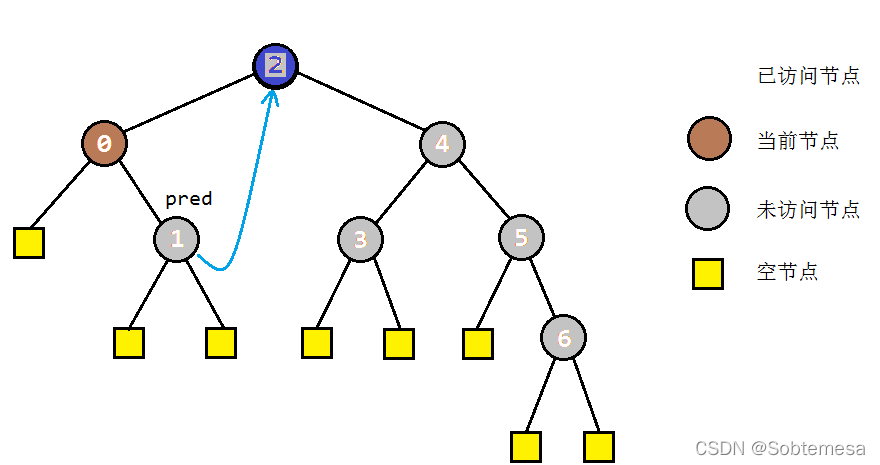
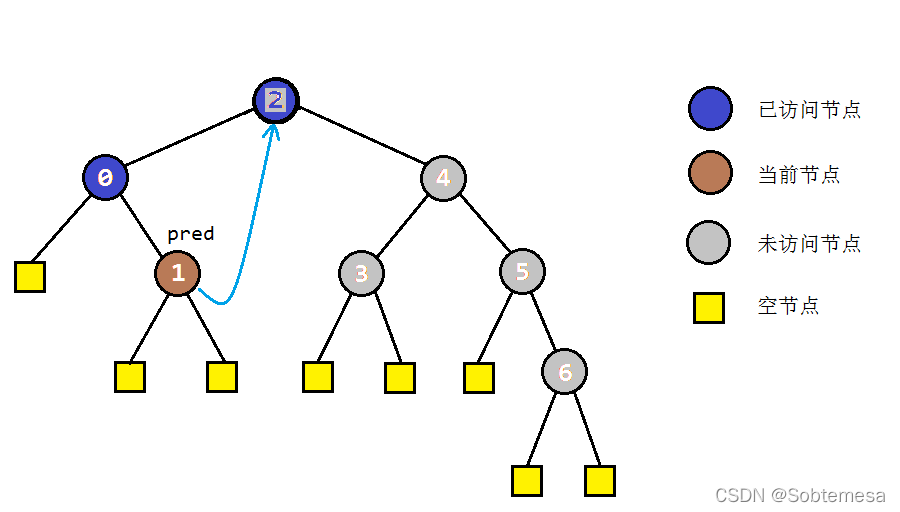
在这里插入图片描述

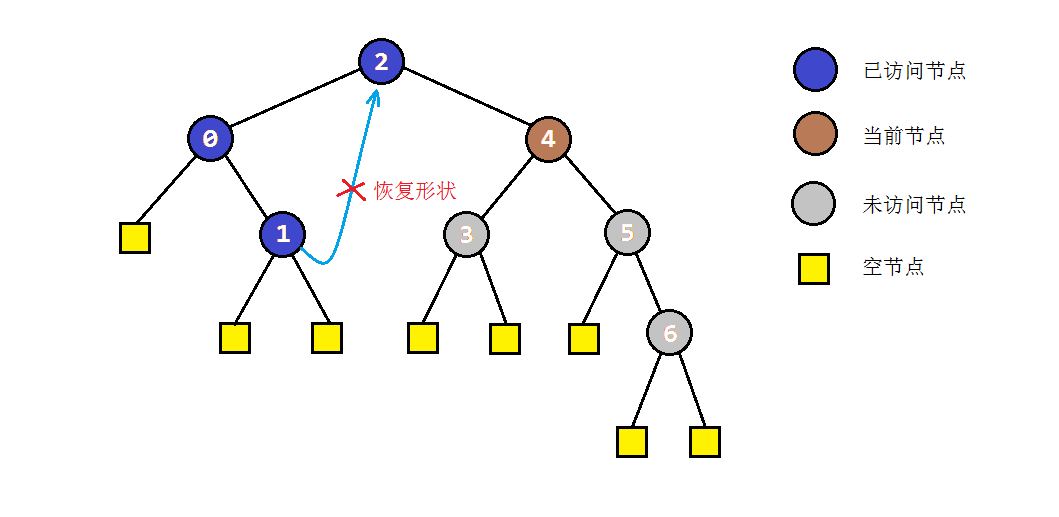
Morris遍历过程5.png

cur依次遍历的序列叫做Morris序，上图的Morris序列为：2 0 1 2 4 3 4 5 6

1）如果节点没有左树，遍历时只到达一次。

2）如果节点有左树，遍历时到达两次。

3）节点在第二次到达之前会遍历完此节点的左树。

4）Morris遍历用节点左子树最右节点右指针的指向来标记第几次来到此节点。

? a:如果最右节点右指针指向为空，则为第一次来到此节点。

? b:如果最右节点右指针指向为此节点，则为第二次来到此节点。

判断前中后序：

1）前序遍历：第一次来到此节点，打印。则为：2 0 1 4 3 5 6

代码：

 /**
    * 前序遍历morris
    * * @param root
    * @return
    */
   public void preOrder4(BTNode root){
      BTNode pred=null;
      BTNode cur=root;
      if(root==null) return;
      while (cur!=null){
         pred=cur.left;
         //cur有左子树，能来到此节点二次
         if(pred!=null){
            //寻找此节点的前驱节点，即其左子树上的最右节点pred
           while (pred.right!=null&&pred.right!=cur){
              pred=pred.right;
           }
           //第一次来到此节点
           if(pred.right==null){
              System.out.print(cur.val+"->");
              pred.right=cur;
              cur=cur.left;
              continue;
           }
           //第二次来到此节点，说明此节点的左子树都已经遍历过了
           else {
              pred.right=null;
              cur=cur.right;
           }
         }
         //cur没有左子树，只来到此节点一次
         else {
            System.out.print(cur.val+"->");
            cur=cur.right;
         }
      }
      System.out.println();
   }

2）中序遍历：0 1 2 3 4 5 6

? a:如果节点有左树，遍历时到达两次，第二次来到此节点时打印。

? b: 如果节点没有左树，遍历时只到达一次,第一次来到此节点时打印。

代码：

  /**
    * 中序遍历morris
    * @param root
    */
   public void inOrder4(BTNode root){
      BTNode pred=null;
      BTNode cur=root;
      if(root==null) return;
      while (cur!=null){
         pred=cur.left;
         //cur有左子树，能来到此节点二次
         if(pred!=null){
            //寻找此节点的前驱节点，即其左子树上的最右节点pred
            while (pred.right!=null&&pred.right!=cur){
               pred=pred.right;
            }
            //第一次来到此节点
            if(pred.right==null){

               pred.right=cur;
               cur=cur.left;
               continue;
            }
            //第二次来到此节点，说明此节点的左子树都已经遍历过了
            else {
               System.out.print(cur.val+"->");
               pred.right=null;
               cur=cur.right;
            }
         }
         //cur没有左子树，只来到此节点一次
         else {
            System.out.print(cur.val+"->");
            cur=cur.right;
         }
      }
      System.out.println();
   }

3）后序遍历：1 0 3 6 5 4 2

? 第一步：找能遍历到两次的节点，逆序打印该节点左树右边界。

? 第二步：逆序打印整棵树的右边界。

    /**
    * 后序遍历morris
    * @param root
    */
   public void postOrder4(BTNode root){
      BTNode pred=null;
      BTNode cur=root;
      if(root==null) return;
      while (cur!=null){
         pred=cur.left;
         //cur有左子树，能来到此节点二次
         if(pred!=null){
            //寻找此节点的前驱节点，即其左子树上的最右节点pred
            while (pred.right!=null&&pred.right!=cur){
               pred=pred.right;
            }
            //第一次来到此节点
            if(pred.right==null){

               pred.right=cur;
               cur=cur.left;
               continue;
            }
            //第二次来到此节点，说明此节点的左子树都已经遍历过了
            else {

               pred.right=null;
               print(cur.left);
               cur=cur.right;
            }
         }
         //cur没有左子树，只来到此节点一次
         else {

            cur=cur.right;
         }
      }
      print(root);

   }
   //逆序打印出此节点左子树的右边界，打印完要逆置回来
   public void print(BTNode head){
      BTNode tail=reverseEdge(head);
      BTNode cur=tail;
      while (cur!=null){
         System.out.print(cur.val+"->");

         cur=cur.right;
      }

      reverseEdge(tail);
   }
   //类似于单链表的逆置
   public BTNode reverseEdge(BTNode head){
      BTNode pre=null;
      BTNode next=null;
      while (head!=null){
         next=head.right;
         head.right=pre;

         pre=head;
         head=next;

      }
      return pre;
   }