[数据结构与算法]leecode-C语言实现-4. 寻找两个正序数组的中位数

一、题目


二、解题思路
（1）思路一
由于两个数组是有序的，所以可以用插入排序或qsort方法进行数组排序合并，排完序之后，如果元素总个数n为奇数，中位数为第（n-1）/2+1个元素（不是索引位）。如果元素总个数n为偶数，中位数为第n/2+1个和第n/2个的和除以二，再根据中位数的位置在排好序的数组中遍历找出。
此思路没有用代码进行实现，由于此思路的算法复杂度为O(n^2),并且需要重新定义一个长度为m+n的数组，占用的内存会比较多。

（2）思路二
先找出中位数的位置，如果元素总个数n为奇数，中位数为第（n-1）/2+1个元素（不是索引位）。如果元素总个数n为偶数，中位数为第n/2+1个和第n/2个的和除以二。我们其实不需要用额外的数组来记录元素，只需要按照情况来划分，建议看到下面提供的代码，再对着思路二一起看，对理解有帮助。

这里需要用到一些变量来协助
int flg;
1表示元素个数为偶数
0表示元素个数为奇数

int cnt;
记录遍历了多少个元素

int ResIndex[2];
记录中位数的位置，不是记录索引号

int NumExsits(int num, int *arr)
判断num是否在数组arr中，在的话返回索引位，不在返回-1

int tmp = -1;
将NumExsits的结果赋予tmp

int twoflg = 0;
表示中位数已经取几个

情况一：两个数组同时遍历，得到了中位数：

1、数组A中的元素a大于数组B中的元素a，是否到中位数记录的位置，在的话，再判断总元素个数是奇数，直接返回中位数，如果是偶数，表示中位数是两个数的和，再判断twoflg为几，如果为1，表示之前已经取了一个，将此元素加到结果中并返回。如果为0，将此元素加到结果中继续循环。

2、数组A中的元素a小于数组B中的元素a，逻辑同上。

3、数组A中的元素a等于数组B中的元素a，是否到中位数记录的位置，在的话，再判断总元素个数是奇数，直接返回中位数，如果是偶数，表示中位数是两个数的和，再判断twoflg为几，如果为1，且结果值中为0，表示这两个元素为所求的中位数。如果res结果不为0，表示之前已经存储了一个元素，最多存储两个元素，所以直接把元素加到结果中返回。再者就是结果值为0，但中位数需要两个数才能确认的情况，把元素加到结果中继续循环。

情况二：两个数组同时遍历，没有得到中位数：
这种情况表示其中一个数组已经完全遍历完毕，只需遍历其中一个数组即可，我这里两个都写是因为其中有一个循环必然不会进入，这样不用多加判断，代码简洁一些。

1、元素个数为奇数，中位数为一个的时候，上面的情况没有过滤到元素，在数组中遍历，遍历到直接返回结果。

2、元素个数为偶数，中位数为两个的时候，上面的情况没有过滤到元素，在数组中遍历，遍历到两次，直接将结果相加再除以二返回结果即可。

3、元素个数为偶数，中位数为两个的时候，上面的情况过滤到一个元素，在数组中遍历，遍历到一次，直接将结果相加再除以二返回结果即可。

三、虚机测试代码

#include <stdio.h>
#include <stdlib.h>

void main()
{
    void PrintfArr(void *arr, int size ,int elementsize);
    double findMedianSortedArrays(int* nums1, int nums1Size, int* nums2, int nums2Size);

    //int nums1[] = {1,2,4,5,6};
    //int nums2[] = {3,5,6,7,8,9,10,11,12,13};
    //int nums2[] = {3,5,6,7,8,9,10,11,12,13,14,15};
    //int nums2[] = {3,5,6,7,8,9,10,11,12,13,14,15,16};

    int nums1[] = {2,2,4,4};
    int nums2[] = {2,2,4,4};
    int nums1Size = sizeof(nums1) / sizeof(int);
    int nums2Size = sizeof(nums2) / sizeof(int);

    PrintfArr(nums1, nums1Size ,sizeof(int));
    PrintfArr(nums2, nums2Size ,sizeof(int));
    findMedianSortedArrays(nums1,  nums1Size, nums2, nums2Size);
}

double findMedianSortedArrays(int* nums1, int nums1Size, int* nums2, int nums2Size)
{
    void PrintfArr(void *arr, int size ,int elementsize);
    int NumExsits(int num, int *arr);
    int ResIndex[2];
    int flg;

    if((nums1Size + nums2Size) % 2 == 0)
    {
        ResIndex[0] = (nums1Size + nums2Size) / 2;
        ResIndex[1] = (nums1Size + nums2Size) / 2 + 1;
        flg = 1;
    }
    else
    {
        ResIndex[0] = ((nums1Size + nums2Size) - 1) / 2 + 1;
        ResIndex[1] = -1;    
        flg = 0;
    }
    PrintfArr(ResIndex,2,sizeof(int));

    int x,y,cnt;
    double res = 0;
    int tmp = -1;
    int twoflg = 0;
    for(x=0,y=0,cnt=0; x<nums1Size && y<nums2Size;)
    {
        cnt++;
        if(nums1[x] < nums2[y] && x<nums1Size && y<nums2Size)
        {
            tmp = NumExsits(cnt, ResIndex);
            printf("cnt : %d, tmp : %2d, flg : %d, nums1[%d] : %d, nums2[%d] : %d, twoflg : %d\n",cnt,tmp,flg,x,nums1[x],y,nums2[y],twoflg);
            printf("nums1[x] < nums2[y]\n");
            if(tmp != -1)
            {
                if(!flg)
                {
                    res = res + nums1[x];
                    printf("res : %f\n",res);
                    return res;
                }
                else if(twoflg == 1)
                {
                    res = (res + nums1[x]) / 2;
                    printf("res : %f\n",res);
                    return res;
                }
                else
                {   
                    res = res + nums1[x];
                    printf("res : %f\n",res);
                    twoflg++;
                }
            }
            x++;
        }
        else if(nums1[x] > nums2[y] && x<nums1Size && y<nums2Size)
        {
            tmp = NumExsits(cnt, ResIndex);
            printf("cnt : %d, tmp : %2d, flg : %d, nums1[%d] : %d, nums2[%d] : %d, twoflg : %d\n",cnt,tmp,flg,x,nums1[x],y,nums2[y],twoflg);
            printf("nums1[x] > nums2[y]\n");   
            if(tmp != -1)
            {   
                if(!flg)
                {
                    res = res + nums2[y];
                    printf("res : %f\n",res);
                    return res;   
                }
                else if(twoflg == 1)
                {   
                    res = (res + nums2[y]) / 2;
                    printf("res : %f\n",res);
                    return res;
                }
                else
                {
                    res = res + nums2[y];
                    printf("res : %f\n",res);
                    twoflg++;
                }
            }
            y++;
        }
        else if(nums1[x] == nums2[y] && x<nums1Size && y<nums2Size)
        {
            tmp = NumExsits(cnt, ResIndex);
            if(tmp > -1)
            {
                twoflg++;
            }
            cnt++;
            tmp = NumExsits(cnt, ResIndex);
            if(tmp > -1)
            {
                twoflg++;
            }
            printf("cnt : %d, tmp : %2d, flg : %d, nums1[%d] : %d, nums2[%d] : %d, twoflg : %d\n",cnt,tmp,flg,x,nums1[x],y,nums2[y],twoflg);
            printf("nums1[x] == nums2[y]\n");   
            if(twoflg > 0)
            {   
                if(flg == 0)
                {
                    res = res + nums1[x];
                    printf("res : %f\n",res);
                    return res;   
                }
                else if(flg && twoflg == 2 && res == 0)
                {   
                    res = (res + nums1[x] + nums2[y]) / 2;
                    printf("res : %f\n",res);
                    return res;
                }
                else if(res != 0)
                {
                    res = (res + nums1[x]) / 2;
                    printf("res : %f\n",res);    
                    return res;
                }
                else
                {
                    res = res + nums1[x];
                    printf("res : %f\n",res);
                }
            }
            x++;
            y++;
            if(twoflg == 2)
            {
                twoflg = 0;
            }
        }
        printf("++++++++++++++++++++\n");
    }

    for(; x<nums1Size; x++)
    {
        cnt++;
        tmp = NumExsits(cnt, ResIndex);
        if(tmp > -1 && !flg)
        {
            res = res + nums1[x];
            printf("res : %f\n",res);
            return res;
        }
        else if(tmp > -1 && flg && twoflg == 1)
        {
            res = (res + nums1[x]) / 2;
            printf("res : %f\n",res);
            return res;
        }
        else if(tmp > -1 && flg && twoflg == 0)
        {
            res = res + nums1[x];
            printf("res : %f\n",res);
            twoflg++;
        }        
    }
    for(; y<nums2Size; y++)
    {
        cnt++;
        tmp = NumExsits(cnt, ResIndex);
        if(tmp > -1 && !flg)
        {   
            res = res + nums2[y];
            printf("res : %f\n",res);
            return res;
        }
        else if(tmp > -1 && flg && twoflg == 1)
        {   
            res = (res + nums2[y]) / 2;
            printf("res : %f\n",res);
            return res;
        }
        else if(tmp > -1 && flg && twoflg == 0)
        {   
            res = res + nums2[y];
            printf("res : %f\n",res);
            twoflg++;
        }    
    }
}

int NumExsits(int num, int *arr)
{
    int i;
    for(i=0; i<2; i++)
    {
        if(arr[i] == num)
        {
            return i;
        }
    }
    return -1;
}

void PrintfArr(void *arr, int size ,int elementsize)
{
    if(elementsize == sizeof(int))
    {
        int *tmparr = (int*)arr;
        int i;
        for(i=0; i<size; i++)
        {
            printf("%d ",tmparr[i]);
        }
    }
    else if(elementsize == sizeof(char))
    {
        char *tmparr = (char*)arr;
        int i;
        for(i=0; i<size; i++)
        {
            printf("%c ",tmparr[i]);
        }
    }
    printf("\n");
}

四、虚机测试截图

五、leecode提交代码

double findMedianSortedArrays(int* nums1, int nums1Size, int* nums2, int nums2Size)
{
    //void printfArr(void *arr, int size ,int elementsize);
    int NumExsits(int num, int *arr);
    int ResIndex[2];
    int flg;

    if((nums1Size + nums2Size) % 2 == 0)
    {
        ResIndex[0] = (nums1Size + nums2Size) / 2;
        ResIndex[1] = (nums1Size + nums2Size) / 2 + 1;
        flg = 1;
    }
    else
    {
        ResIndex[0] = ((nums1Size + nums2Size) - 1) / 2 + 1;
        ResIndex[1] = -1;    
        flg = 0;
    }
    //printfArr(ResIndex,2,sizeof(int));

    int x,y,cnt;
    double res = 0;
    int tmp = -1;
    int twoflg = 0;
    for(x=0,y=0,cnt=0; x<nums1Size && y<nums2Size;)
    {
        cnt++;
        if(nums1[x] < nums2[y] && x<nums1Size && y<nums2Size)
        {
            tmp = NumExsits(cnt, ResIndex);
            //printf("cnt : %d, tmp : %2d, flg : %d, nums1[%d] : %d, nums2[%d] : %d, twoflg : %d\n",cnt,tmp,flg,x,nums1[x],y,nums2[y],twoflg);
            //printf("nums1[x] < nums2[y]\n");
            if(tmp != -1)
            {
                if(!flg)
                {
                    res = res + nums1[x];
                    //printf("res : %f\n",res);
                    return res;
                }
                else if(twoflg == 1)
                {
                    res = (res + nums1[x]) / 2;
                    //printf("res : %f\n",res);
                    return res;
                }
                else
                {   
                    res = res + nums1[x];
                    //printf("res : %f\n",res);
                    twoflg++;
                }
            }
            x++;
        }
        else if(nums1[x] > nums2[y] && x<nums1Size && y<nums2Size)
        {
            tmp = NumExsits(cnt, ResIndex);
            //printf("cnt : %d, tmp : %2d, flg : %d, nums1[%d] : %d, nums2[%d] : %d, twoflg : %d\n",cnt,tmp,flg,x,nums1[x],y,nums2[y],twoflg);
            //printf("nums1[x] > nums2[y]\n");   
            if(tmp != -1)
            {   
                if(!flg)
                {
                    res = res + nums2[y];
                    //printf("res : %f\n",res);
                    return res;   
                }
                else if(twoflg == 1)
                {   
                    res = (res + nums2[y]) / 2;
                    //printf("res : %f\n",res);
                    return res;
                }
                else
                {
                    res = res + nums2[y];
                    //printf("res : %f\n",res);
                    twoflg++;
                }
            }
            y++;
        }
        else if(nums1[x] == nums2[y] && x<nums1Size && y<nums2Size)
        {
            tmp = NumExsits(cnt, ResIndex);
            if(tmp > -1)
            {
                twoflg++;
            }
            cnt++;
            tmp = NumExsits(cnt, ResIndex);
            if(tmp > -1)
            {
                twoflg++;
            }
            //printf("cnt : %d, tmp : %2d, flg : %d, nums1[%d] : %d, nums2[%d] : %d, twoflg : %d\n",cnt,tmp,flg,x,nums1[x],y,nums2[y],twoflg);
            //printf("nums1[x] == nums2[y]\n");   
            if(twoflg > 0)
            {   
                if(flg == 0)
                {
                    res = res + nums1[x];
                    //printf("res : %f\n",res);
                    return res;   
                }
                else if(flg && twoflg == 2 && res == 0)
                {   
                    res = (res + nums1[x] + nums2[y]) / 2;
                    //printf("res : %f\n",res);
                    return res;
                }
                else if(res != 0)
                {
                    res = (res + nums1[x]) / 2;
                    //printf("res : %f\n",res);    
                    return res;
                }
                else
                {
                    res = res + nums1[x];
                    //printf("res : %f\n",res);
                }
            }
            x++;
            y++;
            if(twoflg == 2)
            {
                twoflg = 0;
            }
        }
        //printf("++++++++++++++++++++\n");
    }

    for(; x<nums1Size; x++)
    {
        cnt++;
        tmp = NumExsits(cnt, ResIndex);
        if(tmp > -1 && !flg)
        {
            res = res + nums1[x];
            //printf("res : %f\n",res);
            return res;
        }
        else if(tmp > -1 && flg && twoflg == 1)
        {
            res = (res + nums1[x]) / 2;
            //printf("res : %f\n",res);
            return res;
        }
        else if(tmp > -1 && flg && twoflg == 0)
        {
            res = res + nums1[x];
            //printf("res : %f\n",res);
			twoflg++;
        }        
    }
    for(; y<nums2Size; y++)
    {
        cnt++;
        tmp = NumExsits(cnt, ResIndex);
        if(tmp > -1 && !flg)
        {   
            res = res + nums2[y];
            //printf("res : %f\n",res);
            return res;
        }
        else if(tmp > -1 && flg && twoflg == 1)
        {   
            res = (res + nums2[y]) / 2;
            //printf("res : %f\n",res);
            return res;
        }
        else if(tmp > -1 && flg && twoflg == 0)
        {   
            res = res + nums2[y];
            //printf("res : %f\n",res);
			twoflg++;
        }    
    }
	return -1;
}

int NumExsits(int num, int *arr)
{
    int i;
    for(i=0; i<2; i++)
    {
        if(arr[i] == num)
        {
            return i;
        }
    }
    return -1;
}

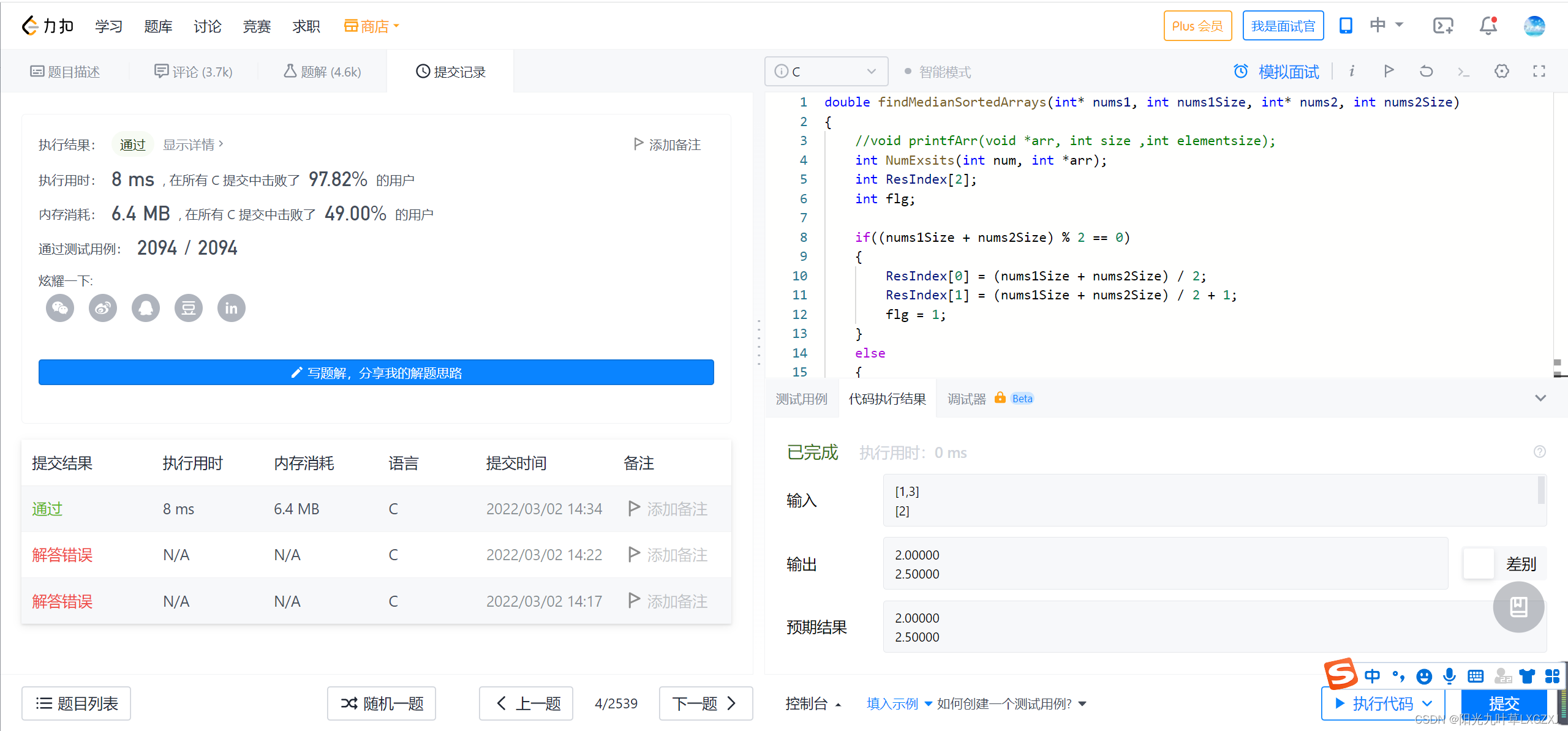
六、leecode代码提交截图