作文以记之 ~ 计算 x 的 n 次幂函数
0、前言
本篇博客是针对力扣上50题的题解,记载了做题过程中的几种做法,有可实现的,也有不可实现的!具体的题目描述可 点击此处 进行查看!具体的代码实现以及其他内容可 点击此处 进行查看!
1、题目描述
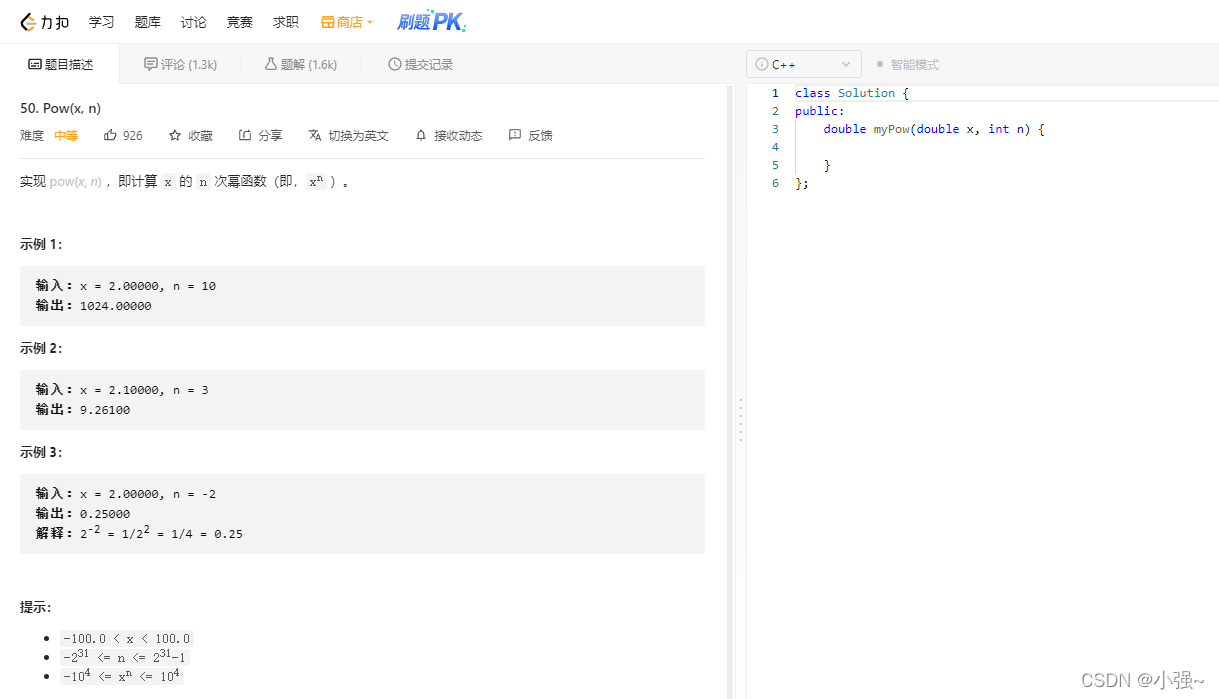
2、解题思路
2.1 方法1 ~ 利用递归
2.1.1 思路
这种思路就是直接利用递归实现计算 x 的 n 次幂函数,但没考虑当幂次特别大时,程序的运行效率,所以在力扣上有些案例就没通过!在这里就是记录一下自己的弱鸡时间~
2.1.2 程序代码
#include<iostream>
#include<vector>
using namespace std;
#include<algorithm>
/* 方法1 ~ 利用递归 (有问题)*/
double ans(double x, int n)
{
if (n < 0)
{
n = -n;
x = 1 / x;
}
if (n < 1)
return 1;
--n;
return ans(x, n)*x;
}
double myPow(double x, int n)
{
return ans(x, n);
}
void test()
{
double x = 2.0; int n = 3;
cout << "\n输入:x = " << x << " n = " << n << endl;
cout << "\n结果:";
cout << myPow(x,n) << endl << endl;
}
int main()
{
test();
system("pause");
return 0;
}
2.1.3 运行结果
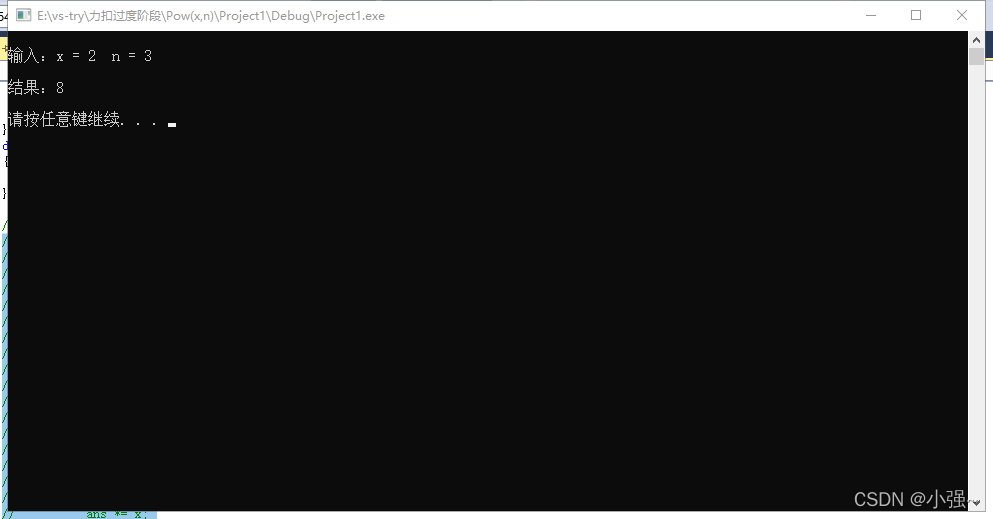
2.2 方法2 ~ 利用遍历
2.2.1 思路
这又是一个菜鸡想法,依然是运行效率慢,力扣案例也有过不了的,害~
2.2.2 程序代码
#include<iostream>
#include<vector>
using namespace std;
#include<algorithm>
/* 方法2 ~ 遍历 (有问题)*/
double myPow(double x, int n) {
double res = 1.0;
if (n < 0) n = -n, x = 1 / x;
for (int i = 0; i < n; i++)
{
res *= x;
}
return res;
}
void test()
{
double x = 2.0; int n = 3;
cout << "\n输入:x = " << x << " n = " << n << endl;
cout << "\n结果:";
cout << myPow(x,n) << endl << endl;
}
int main()
{
test();
system("pause");
return 0;
}
2.2.3 运行结果
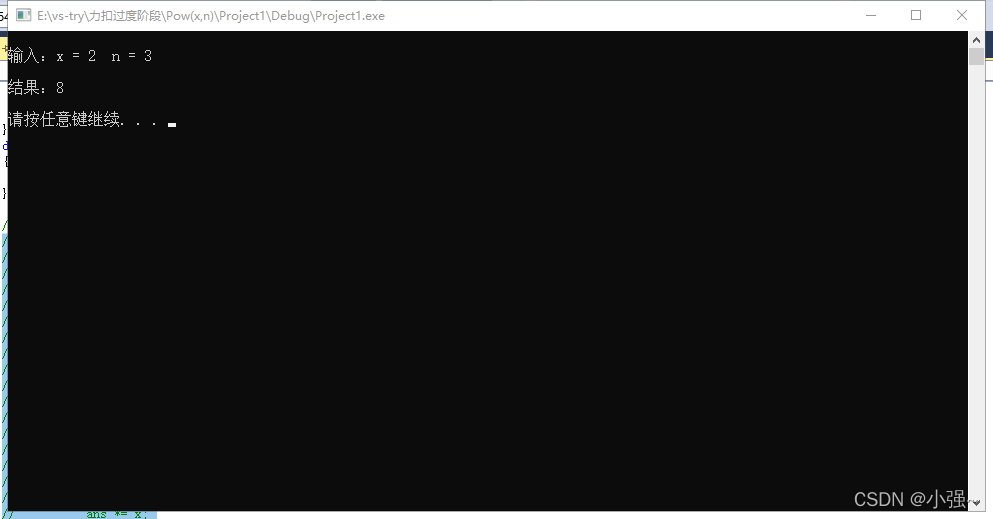
2.3 方法3 ~ 利用快速幂
2.3.1 思路
这种思路就是利用x的平方取进行操作,例如
举个例子,计算 x^32 可以按照:
x -> x ^2 -> x^4 -> x^8 -> x^16 -> x^32 进行操作,即可以将偶数次的直接乘以x的平方,然后奇数次的乘1(n为偶数)或x(n为奇数)!
2.3.2 程序代码
#include<iostream>
#include<vector>
using namespace std;
#include<algorithm>
/* 方法3 ~ 奇数幂 */
double myPow(double x, int n) {
double ans = 1.0;
for (int i = n; i != 0; i /= 2)
{
if (i % 2 != 0)
{
ans *= x;
}
x *= x;
}
return n < 0 ? 1 / ans : ans;
}
void test()
{
double x = 2.0; int n = 3;
cout << "\n输入:x = " << x << " n = " << n << endl;
cout << "\n结果:";
cout << myPow(x,n) << endl << endl;
}
int main()
{
test();
system("pause");
return 0;
}
2.3.3 运行结果
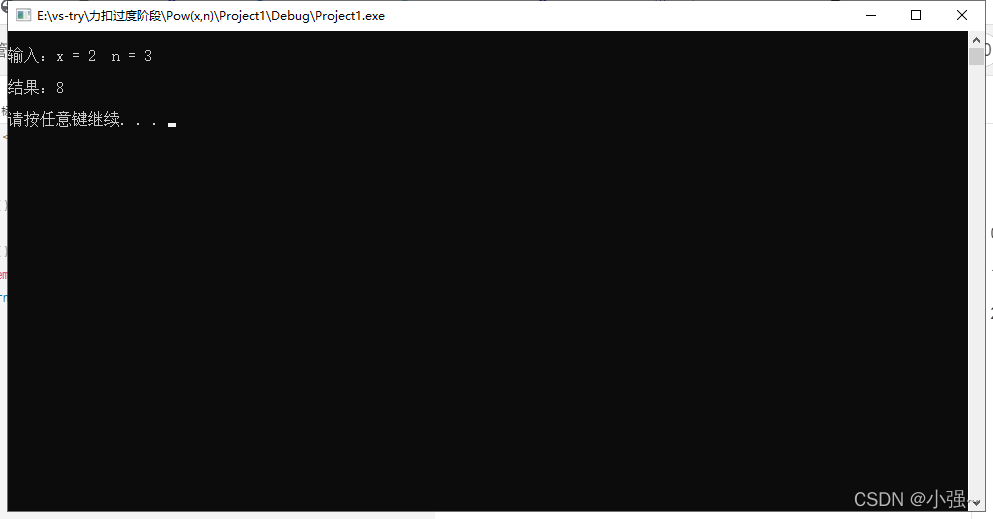
3、总结
写这篇博客主要是觉得这第三种解法有点意思,然后对其进行记录,方便以后学习!
