[数据结构与算法]25.K个一组翻转链表

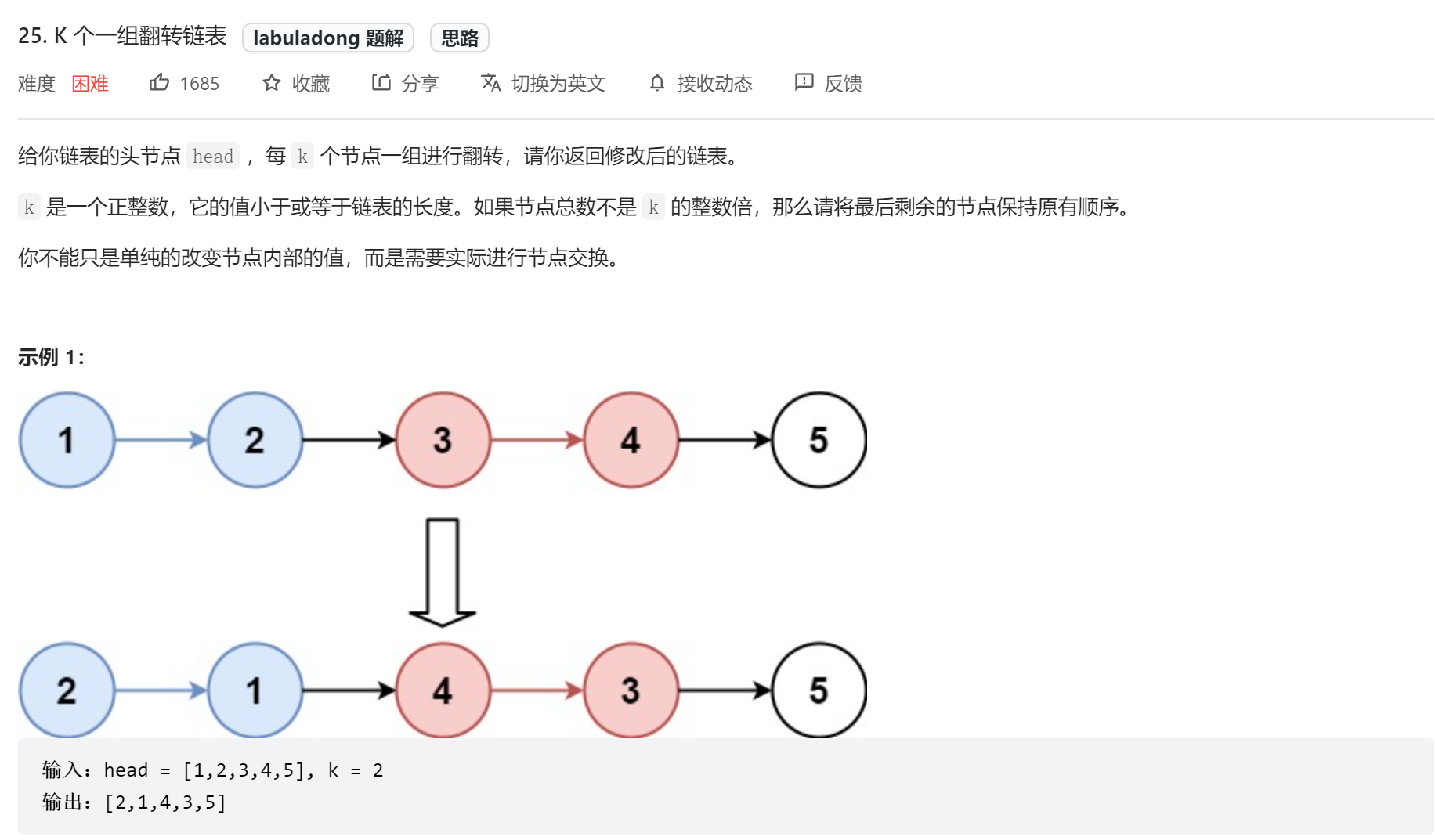
思路一 改变链表方向+分组反转

    class Solution {
        public ListNode reverseKGroup(ListNode head, int k) {

            // 创建保护节点
            ListNode protect = new ListNode(-1, head);
            ListNode last = protect;

            while(head != null) {

                // 获取节点尾部
                // 如果不满足条件, 比如 仅剩2个节点, 但是要返回3组, 就会直接返回null
                ListNode tail = getTail(head, k);

                // 边界问题 : tail = null , 最后一组不满足 k 个
                if (tail == null) {
                    break;
                }

                // 记录尾部节点的下一个节点
                ListNode nextNode = tail.next;

                // 反转 head 到 tail 之间 n - 1 条边
                reverse(head, tail);

                // 上一组的尾部(未反转前的头部)指向当前组的头部(未反转前的尾部)
                last.next = tail;
                head.next = nextNode;

                // 向后移动
                last = head;
                head = nextNode;

            }

            return protect.next;
        }

        public ListNode getTail(ListNode head, int k){

            int walk = k - 1; // 实际要走的步数

            if(walk <= 0) {
                return head;
            }

            for(int i = 0; head != null && i < walk; i++ ) {
                head = head.next;
            }

            return head;
        }

        private void reverse(ListNode head, ListNode tail){

            if (head == tail) {
                return;
            }

            // 这里注意, 我们不需要改变第一个节点的边的指向
            // 所以原先 last = null => last = head , 向后移动一位
            ListNode last = head;
            head = head.next;
            while(head != tail) {
                ListNode nextNode = head.next;
                // 改变边的方向
                head.next = last;
                // 指针向后移动
                last = head;
                head = nextNode;
            }
            // 注意 head 移动到 tail 位置时, 最后一条边的方向还未改变
            tail.next = last;
        }
    }

基本思路如下 :

主体思路很简单, 分组去反转对应部分的链表, 然后再去考虑和当前组前面和后面节点的链接

首先我们要实现一个获取链表尾部的方法 :

• 传入 head 和 链表的个数n 返回链表的尾部

        public ListNode getTail(ListNode head, int k){

            int walk = k - 1; // 实际要走的步数

            if(walk <= 0) {
                return head;
            }

            for(int i = 0; head != null && i < walk; i++ ) {
                head = head.next;
            }

            return head;
        }

代码很简单, 但是要注意一些细节 :

• 我们要从head移动k步到尾部, 所以实际走的步数数 k - 1, 因为默认就在 head 上.
• head != null 这个条件, 当 最后一组的链表节点个数小于 k - 1的时候, 这个时候 还未循环结束, head 可能就走到了链表的末尾, 注意 : 此时 我们要返回的 tail 并不是链表的末尾, 而是 null

其次我们要将 链表的头部和尾部节点传入到 reverse 方法内去反转链表的边, 这个思路和 206.反转链表 的思路基本一致, 但是有一点不同

        private void reverse(ListNode head, ListNode tail){

            if (head == tail) {
                return;
            }

            // 这里注意, 我们不需要改变第一个节点的边的指向
            // 所以原先 last = null => last = head , 向后移动一位
            ListNode last = head;
            head = head.next;
            while(head != tail) {
                ListNode nextNode = head.next;
                // 改变边的方向
                head.next = last;
                // 指针向后移动
                last = head;
                head = nextNode;
            }
            // 注意 head 移动到 tail 位置时, 最后一条边的方向还未改变
            tail.next = last;
        }

注意细节 :

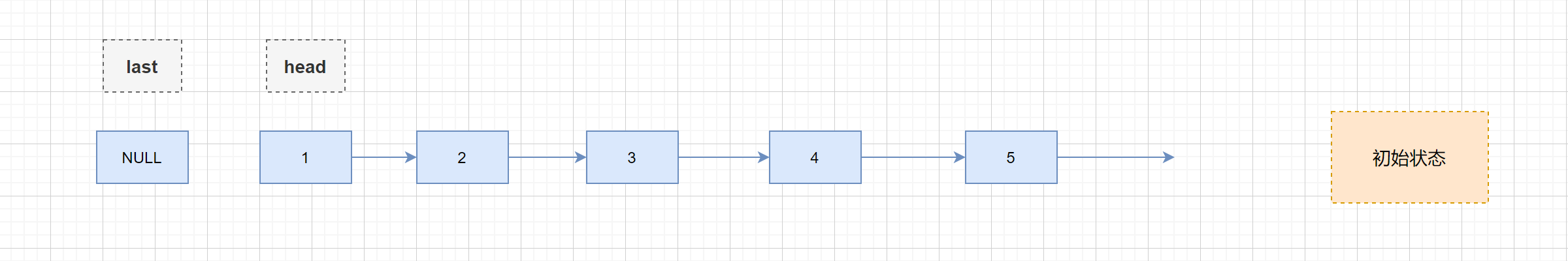
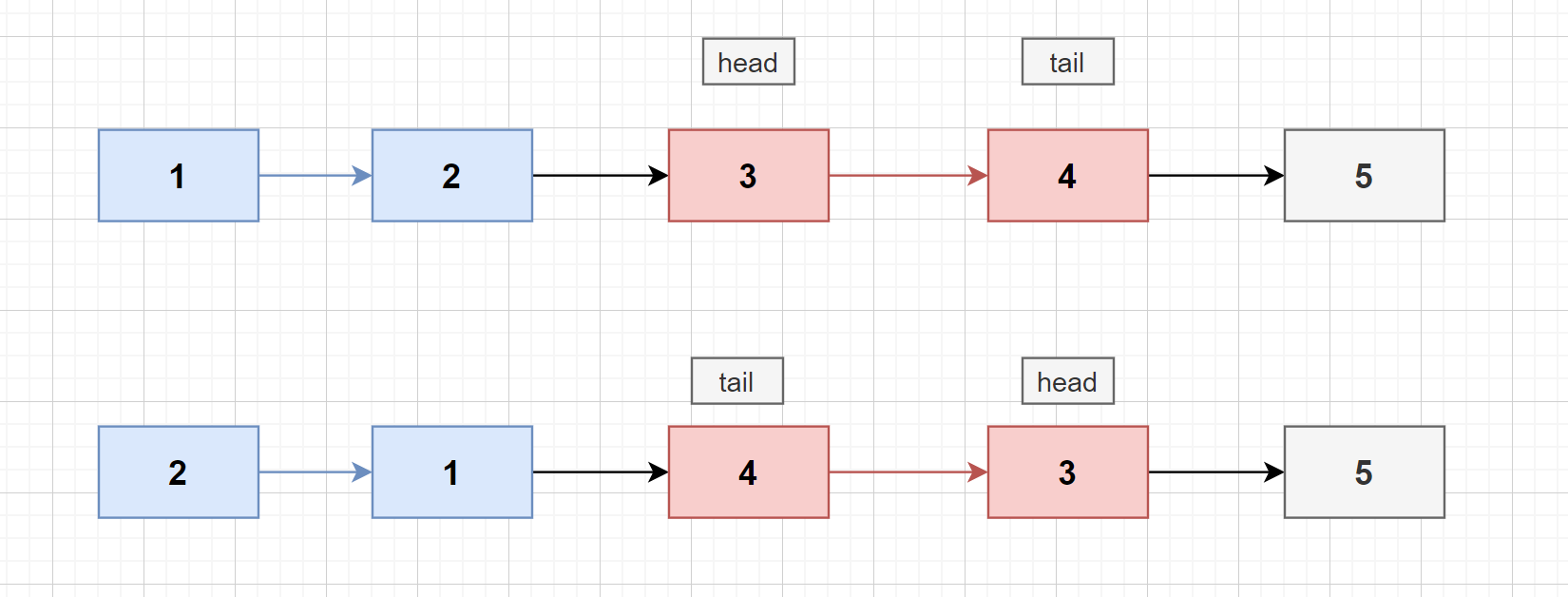
反转链表时, 初始状态 last 是指向 null 的, 假如有5个节点, 我们实际上要反转 5条边, 包含开头指向null的边

但是在这道题里面, 我们仅仅需要反转 n - 1 条边,如下图, 实际上我们要反转的是 节点 1 到 节点 2 的边即可

细节问题: 如果head和tial指向同一个节点, 我们就不需要反转边

   if (head == tail) {
       return;
   }

然后, 我们需要把反转后的链表和其他节点连接, 另外需要注意的是, 我们考虑问题先考虑整体, 再考虑细节, 所以我们考虑中间的节点, 因为中间的节点不存在边界问题 :

 public ListNode reverseKGroup(ListNode head, int k) {

            // 创建保护节点
            ListNode protect = new ListNode(-1, head);
            ListNode last = protect;

            while(head != null) {

                // 获取节点尾部
                // 如果不满足条件, 比如 仅剩2个节点, 但是要返回3组, 就会直接返回null
                ListNode tail = getTail(head, k);

                // 边界问题 : tail = null , 最后一组不满足 k 个
                if (tail == null) {
                    break;
                }

                // 记录尾部节点的下一个节点
                ListNode nextNode = tail.next;

                // 反转 head 到 tail 之间 n - 1 条边
                reverse(head, tail);

                // 上一组的尾部(未反转前的头部)指向当前组的头部(未反转前的尾部)
                last.next = tail;
                head.next = nextNode;

                // 向后移动
                last = head;
                head = nextNode;

            }

            return protect.next;
        }

首先在反转链表之前需要记录尾部节点4的下一个节点5 : ListNode nextNode = tail.next; 因为反转以后, 节点 4 和 5之间的连接就断了

其次就是对链表进行反转, 然后将当前组和上一组的尾部与下一组的头部连接 :

• 前一组的尾部节点 1 指向反转后的头部节点 4 (未反转前的尾部节点) : last.next = tail;
• 当前组的尾部节点 3 (未反转前的头部节点) 指向 之前记录的节点 5 head.next = nextNode;

最后我们需要重新修改last节点指向当前组的尾部节点, 修改head节点指向下一组的头部节点

 // 向后移动
 last = head;
  head = nextNode;

另外注意细节问题: 最后一组不满足k个的时候, 我们不需要反转, 所以当我们获取尾部以后 需要判断下

   // 边界问题 : tail = null , 最后一组不满足 k 个
                if (tail == null) {
                    break;
                }

其次是保护节点 : 之所以要有保护节点的原因是, 我们考虑问题是考虑中间组的节点, 但是对于头结点来说, 它没有上一组的节点, 且无法获取 last (也就是上一组的尾结点) , 但是保护节点就可以很好的解决问题 :

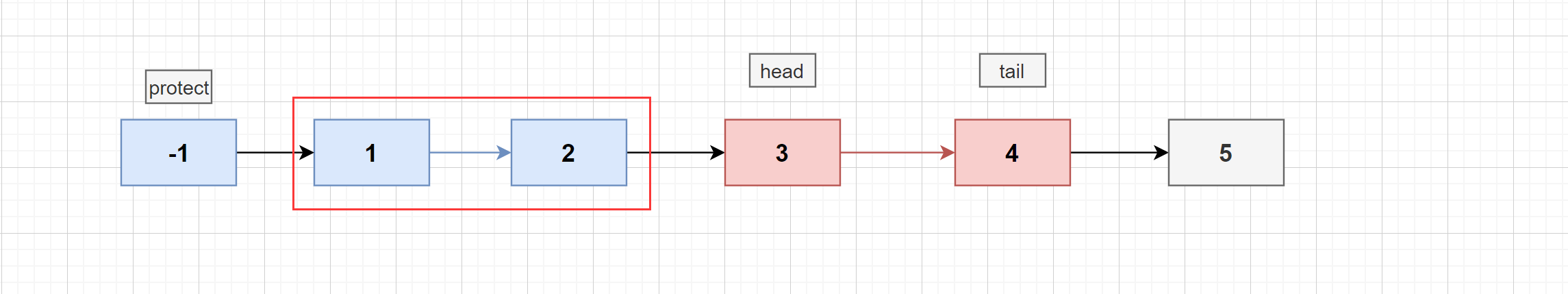
初始时 last 直接指向 protect 节点即可, 头结点也不需要考虑边界问题.

完整代码如下 :

package cn.knightzz.leetcode.hard;

import cn.knightzz.link.ListNode;
import static cn.knightzz.link.utils.ListNodeUtils.*;

@SuppressWarnings("all")
public class LeetCode25 {

    class Solution {
        public ListNode reverseKGroup(ListNode head, int k) {

            // 创建保护节点
            ListNode protect = new ListNode(-1, head);
            ListNode last = protect;

            while(head != null) {

                // 获取节点尾部
                // 如果不满足条件, 比如 仅剩2个节点, 但是要返回3组, 就会直接返回null
                ListNode tail = getTail(head, k);

                // 边界问题 : tail = null , 最后一组不满足 k 个
                if (tail == null) {
                    break;
                }

                // 记录尾部节点的下一个节点
                ListNode nextNode = tail.next;

                // 反转 head 到 tail 之间 n - 1 条边
                reverse(head, tail);

                // 上一组的尾部(未反转前的头部)指向当前组的头部(未反转前的尾部)
                last.next = tail;
                head.next = nextNode;

                // 向后移动
                last = head;
                head = nextNode;

            }

            return protect.next;
        }

        public ListNode getTail(ListNode head, int k){

            int walk = k - 1; // 实际要走的步数

            if(walk <= 0) {
                return head;
            }

            for(int i = 0; head != null && i < walk; i++ ) {
                head = head.next;
            }

            return head;
        }

        private void reverse(ListNode head, ListNode tail){

            if (head == tail) {
                return;
            }

            // 这里注意, 我们不需要改变第一个节点的边的指向
            // 所以原先 last = null => last = head , 向后移动一位
            ListNode last = head;
            head = head.next;
            while(head != tail) {
                ListNode nextNode = head.next;
                // 改变边的方向
                head.next = last;
                // 指针向后移动
                last = head;
                head = nextNode;
            }
            // 注意 head 移动到 tail 位置时, 最后一条边的方向还未改变
            tail.next = last;
        }
    }
}

思路二 计算链表长度+递归法反转

这个方法的思路很简单 : 先计算链表长度, 然后和 k 求余得到总共要反转多少组, 然后分组翻转即可

时间复杂度 $O_n$

• 实现反转前n项的方法 reverseN(ListNode head, int n)
• 实现反转第n到m个元素的方法 : reverseBetwwen(ListNode head, int n , int m)
• 计算链表长度, 然后通过求余得到反转的组数

/**
 * Definition for singly-linked list.
 * public class ListNode {
 *     int val;
 *     ListNode next;
 *     ListNode() {}
 *     ListNode(int val) { this.val = val; }
 *     ListNode(int val, ListNode next) { this.val = val; this.next = next; }
 * }
 */
    class Solution {
        public ListNode reverseKGroup(ListNode head, int k) {

            int count = 1;
            ListNode p = head;

            while(p.next != null) {
                p = p.next;
                count++;
            }

            int nums = count / k;

            int left = 1;
            int right = k;
            ListNode node = head;
            for (int i = 0; i < nums; i++) {
                node = reverseBetween(node, left, right);
                left += k;
                right+= k;
            }
            return node;
        }

        public ListNode reverseBetween(ListNode node, int  left, int right) {

            if (node == null || node.next == null || left >= right) {
                return node;
            }

            int count = left;
            // 创建虚拟头节点 [关键]
            ListNode pre = new ListNode(-1, node);
            ListNode p = pre;

            while(count != 1) {
                p = p.next;
                count--;
            }

            ListNode head = reverseN(p.next,right - left + 1);
            p.next = head;

            return pre.next;
        }

        public ListNode reverseN(ListNode head, int n){

            // 边界条件
            if (n == 1) {
                return head;
            }

            // 翻后转链表的头部
            ListNode tail = reverseN(head.next, n - 1);
            // 翻转链表的头部 , 注意 tail.next = null / 第 n + 1 个节点
            ListNode p = head.next;

            // 原因是 如果顺序反了, tail指向的目标会丢失
            head.next = p.next;
            p.next = head;
            return tail;
        }

    }