[数据结构与算法]Java-数据结构-二叉树＜一＞

一. 二叉树的简单介绍

????????二叉树（Binary tree）是树形结构的一个重要类型。许多实际问题抽象出来的数据结构往往是二叉树形式，即使是一般的树也能简单地转换为二叉树，而且二叉树的存储结构及其算法都较为简单，因此二叉树显得特别重要。二叉树特点是每个节点最多只能有两棵子树，且有左右之分 。

? ? ? ? 其中，满二叉树和完全二叉树是其中比较特殊的类型。如果一棵二叉树只有度为0的节点和度为2的节点，并且度为0的节点在同一层上，则这棵二叉树为满二叉树。深度为k，有n个节点的二叉树当且仅当其每一个节点都与深度为k的满二叉树中编号从1到n的节点一一对应时，称为完全二叉树，完全二叉树的特点是叶子节点只可能出现在层序最大的两层上，即缺失的叶子节点只能在最后一层的叶子节点上。

二. 二叉树的典型代码实现

  public class TreeNode {
      int val;
      TreeNode left;
      TreeNode right;
      TreeNode() {}
      TreeNode(int val) { this.val = val; }
      TreeNode(int val, TreeNode left, TreeNode right) {
          this.val = val;
          this.left = left;
          this.right = right;
      }
 }

三. 二叉树的遍历

? ? ? ? 二叉树的遍历一般有三种方式，递归方式，使用栈和Morris 遍历。递归的方式实现较为简单，栈则是显示维护一个空间，Morris 遍历的核心思想是利用树的大量空闲指针，实现空间开销的极限缩减。

前序遍历

递归

class Solution {
    List<Integer> list= new  ArrayList<>();
    public List<Integer> preorderTraversal(TreeNode root) {
        dfs(root);
        return list;
    }
    public void dfs(TreeNode root){
        if(root == null) return;
        list.add(root.val);
        dfs(root.left);
        dfs(root.right);
    }
}

栈?

class Solution {
    public List<Integer> preorderTraversal(TreeNode root) {
        List<Integer> res = new ArrayList<Integer>();
        if (root == null) {
            return res;
        }

        Deque<TreeNode> stack = new LinkedList<TreeNode>();
        TreeNode node = root;
        while (!stack.isEmpty() || node != null) {
            while (node != null) {
                res.add(node.val);
                stack.push(node);
                node = node.left;
            }
            node = stack.pop();
            node = node.right;
        }
        return res;
    }
}

?Morris 遍历

class Solution {
    public List<Integer> preorderTraversal(TreeNode root) {
        List<Integer> res = new ArrayList<Integer>();
        if (root == null) {
            return res;
        }

        TreeNode p1 = root, p2 = null;

        while (p1 != null) {
            p2 = p1.left;
            if (p2 != null) {
                while (p2.right != null && p2.right != p1) {
                    p2 = p2.right;
                }
                if (p2.right == null) {
                    res.add(p1.val);
                    p2.right = p1;
                    p1 = p1.left;
                    continue;
                } else {
                    p2.right = null;
                }
            } else {
                res.add(p1.val);
            }
            p1 = p1.right;
        }
        return res;
    }
}

中序遍历

递归

class Solution {
    public List<Integer> inorderTraversal(TreeNode root) {
        List<Integer> res = new ArrayList<Integer>();
        inorder(root, res);
        return res;
    }

    public void inorder(TreeNode root, List<Integer> res) {
        if (root == null) {
            return;
        }
        inorder(root.left, res);
        res.add(root.val);
        inorder(root.right, res);
    }
}

栈/迭代

class Solution {
    public List<Integer> inorderTraversal(TreeNode root) {
        List<Integer> res = new ArrayList<Integer>();
        Deque<TreeNode> stk = new LinkedList<TreeNode>();
        while (root != null || !stk.isEmpty()) {
            while (root != null) {
                stk.push(root);
                root = root.left;
            }
            root = stk.pop();
            res.add(root.val);
            root = root.right;
        }
        return res;
    }
}

?Morris

class Solution {
    public List<Integer> inorderTraversal(TreeNode root) {
        List<Integer> res = new ArrayList<Integer>();
        TreeNode predecessor = null;

        while (root != null) {
            if (root.left != null) {
                // predecessor 节点就是当前 root 节点向左走一步，然后一直向右走至无法走为止
                predecessor = root.left;
                while (predecessor.right != null && predecessor.right != root) {
                    predecessor = predecessor.right;
                }
                
                // 让 predecessor 的右指针指向 root，继续遍历左子树
                if (predecessor.right == null) {
                    predecessor.right = root;
                    root = root.left;
                }
                // 说明左子树已经访问完了，我们需要断开链接
                else {
                    res.add(root.val);
                    predecessor.right = null;
                    root = root.right;
                }
            }
            // 如果没有左孩子，则直接访问右孩子
            else {
                res.add(root.val);
                root = root.right;
            }
        }
        return res;
    }
}

后序遍历

递归

class Solution {
    public List<Integer> postorderTraversal(TreeNode root) {
        List<Integer> res = new ArrayList<Integer>();
        postorder(root, res);
        return res;
    }

    public void postorder(TreeNode root, List<Integer> res) {
        if (root == null) {
            return;
        }
        postorder(root.left, res);
        postorder(root.right, res);
        res.add(root.val);
    }
}

栈/迭代

class Solution {
    public List<Integer> postorderTraversal(TreeNode root) {
        List<Integer> res = new ArrayList<Integer>();
        if (root == null) {
            return res;
        }

        Deque<TreeNode> stack = new LinkedList<TreeNode>();
        TreeNode prev = null;
        while (root != null || !stack.isEmpty()) {
            while (root != null) {
                stack.push(root);
                root = root.left;
            }
            root = stack.pop();
            if (root.right == null || root.right == prev) {
                res.add(root.val);
                prev = root;
                root = null;
            } else {
                stack.push(root);
                root = root.right;
            }
        }
        return res;
    }
}

?Morris

class Solution {
    public List<Integer> postorderTraversal(TreeNode root) {
        List<Integer> res = new ArrayList<Integer>();
        if (root == null) {
            return res;
        }

        TreeNode p1 = root, p2 = null;

        while (p1 != null) {
            p2 = p1.left;
            if (p2 != null) {
                while (p2.right != null && p2.right != p1) {
                    p2 = p2.right;
                }
                if (p2.right == null) {
                    p2.right = p1;
                    p1 = p1.left;
                    continue;
                } else {
                    p2.right = null;
                    addPath(res, p1.left);
                }
            }
            p1 = p1.right;
        }
        addPath(res, root);
        return res;
    }

    public void addPath(List<Integer> res, TreeNode node) {
        int count = 0;
        while (node != null) {
            ++count;
            res.add(node.val);
            node = node.right;
        }
        int left = res.size() - count, right = res.size() - 1;
        while (left < right) {
            int temp = res.get(left);
            res.set(left, res.get(right));
            res.set(right, temp);
            left++;
            right--;
        }
    }
}

四. leetcode实战

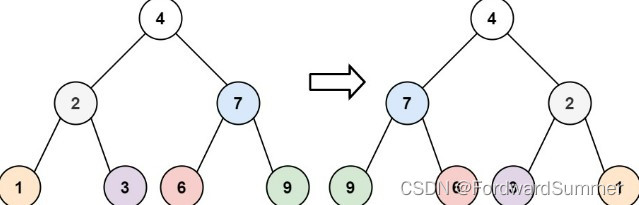
1. leetcode226 翻转二叉树

给你一棵二叉树的根节点 root ，翻转这棵二叉树，并返回其根节点。

输入：root = [4,2,7,1,3,6,9]
输出：[4,7,2,9,6,3,1]

class Solution {
	public TreeNode invertTree(TreeNode root) {
		//递归函数的终止条件，节点为空时返回
		if(root==null) {
			return null;
		}
		//下面三句是将当前节点的左右子树交换
		TreeNode tmp = root.right;
		root.right = root.left;
		root.left = tmp;
		//递归交换当前节点的 左子树
		invertTree(root.left);
		//递归交换当前节点的 右子树
		invertTree(root.right);
		//函数返回时就表示当前这个节点，以及它的左右子树
		//都已经交换完了
		return root;
	}
}

本题小结（1）先在一棵树上操作交换，典型的交换的思想

? ? ? ? ? ? ??（2）然后递归左子树和右子树

2. leetcode104 & leetcode111 二叉树的最大/小深度

????????给定一个二叉树，找出其最大深度。二叉树的深度为根节点到最远叶子节点的最长路径上的节点数。说明: 叶子节点是指没有子节点的节点。

最大深度

class Solution {
    public int maxDepth(TreeNode root) {
        if (root == null) {
            return 0;
        } else {
            int leftHeight = maxDepth(root.left);
            int rightHeight = maxDepth(root.right);
            return Math.max(leftHeight, rightHeight) + 1;
        }
    }
}

最小深度

错误的版本：

class Solution {
    public int minDepth(TreeNode root) {
        if(root == null){
            return 0;
        }else{
            int leftL = minDepth(root.left);
            int rightL = minDepth(root.right);
            return Math.min(leftL,rightL)+1;
        }
    }
}

正确版本：

class Solution {
    public int minDepth(TreeNode root) {
        if(root == null) return 0;
        //这道题递归条件里分为三种情况
        //1.左孩子和有孩子都为空的情况，说明到达了叶子节点，直接返回1即可
        if(root.left == null && root.right == null) return 1;
        //2.如果左孩子和由孩子其中一个为空，那么需要返回比较大的那个孩子的深度        
        int m1 = minDepth(root.left);
        int m2 = minDepth(root.right);
        //这里其中一个节点为空，说明m1和m2有一个必然为0，所以可以返回m1 + m2 + 1;
        if(root.left == null || root.right == null) return m1 + m2 + 1;
        
        //3.最后一种情况，也就是左右孩子都不为空，返回最小深度+1即可
        return Math.min(m1,m2) + 1; 
    }
}

class Solution {
    public int minDepth(TreeNode root) {
        if(root == null) return 0;
        int rightdeep = minDepth(root.right);
        int leftdeep = minDepth(root.left);
        if(root.left ==  null || root.right == null) return rightdeep+leftdeep+1;
        return Math.min(rightdeep,leftdeep)+1;
    }
}

class Solution {
    public int minDepth(TreeNode root) {
        if (root == null) return 0;
        else if (root.left == null) return minDepth(root.right) + 1;
        else if (root.right == null) return minDepth(root.left) + 1;
        else return Math.min(minDepth(root.left), minDepth(root.right)) + 1;
    }
}

?本题小结：（1）这题和求最大值不一样，最大的区别在于若左右节点有一个为空怎么办

参考来源：

【1】leetcode 官方解题 二叉树的前序遍历

【2】leetcode wang_ni_ma 动画演示 两种实现 226. 翻转二叉树?

【3】leetcode?房建斌学算法?二叉树的最小深度-理解递归结束条件

【4】百度百科 二叉树??